Resonator
A resonator is a device or system that exhibits resonance or resonant behavior, that is, it naturally oscillates at some frequencies, called its resonant frequencies, with greater amplitude than at others. The oscillations in a resonator can be either electromagnetic or mechanical (including acoustic). Resonators are used to either generate waves of specific frequencies or to select specific frequencies from a signal. Musical instruments use acoustic resonators that produce sound waves of specific tones.
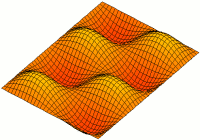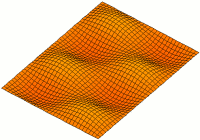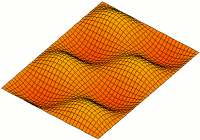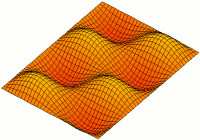
A standing wave in a rectangular cavity resonator
A cavity resonator, usually used in reference to electromagnetic resonators, is one in which waves exist in a hollow space inside the device. Acoustic cavity resonators, in which sound is produced by air vibrating in a cavity with one opening, are known as Helmholtz resonators.
Explanation
A physical system can have as many resonant frequencies as it has degrees of freedom; each degree of freedom can vibrate as a harmonic oscillator. Systems with one degree of freedom, such as a mass on a spring, pendulums, balance wheels, and LC tuned circuits have one resonant frequency. Systems with two degrees of freedom, such as coupled pendulums and resonant transformers can have two resonant frequencies. As the number of coupled harmonic oscillators grows, the time it takes to transfer energy from one to the next becomes significant. The vibrations in them begin to travel through the coupled harmonic oscillators in waves, from one oscillator to the next.
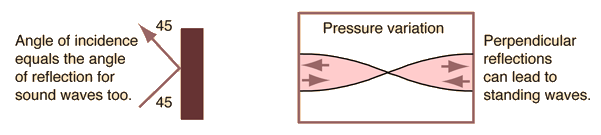
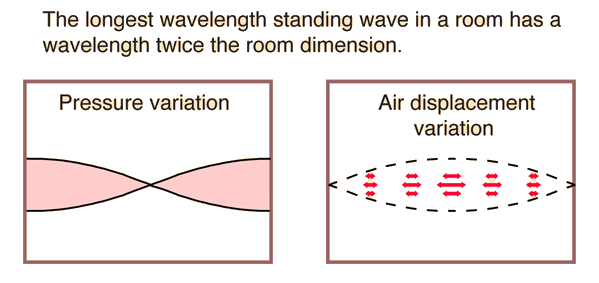
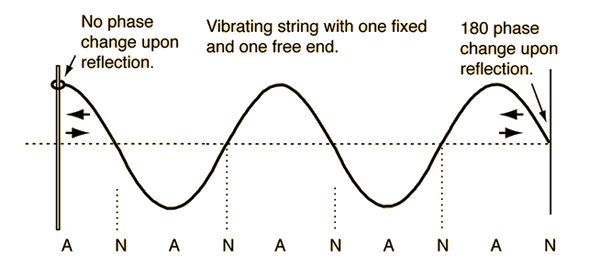
Resonators can be viewed as being made of millions of coupled moving parts (such as atoms). Therefore they can have millions of resonant frequencies, although only a few may be used in practical resonators. The vibrations inside them travel as waves, at an approximately constant velocity, bouncing back and forth between the sides of the resonator. The oppositely moving waves interfere with each other to create a pattern of standing waves in the resonator. If the distance between the sides is

, the length of a round trip is

. In order to cause resonance, the phase of a sinusoidal wave after a round trip has to be equal to the initial phase, so the waves will reinforce. So the condition for resonance in a resonator is that the round trip distance,

, be equal to an integral number of wavelengths
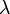
of the wave:

If the velocity of a wave is , the frequency is so the resonance frequencies are:

So the resonant frequencies of resonators, called normal modes, are equally spaced multiples (harmonics), of a lowest frequency called the fundamental frequency. The above analysis assumes the medium inside the resonator is homogeneous, so the waves travel at a constant speed, and that the shape of the resonator is rectilinear. If the resonator is inhomogeneous or has a nonrectilinear shape, like a circular drumhead or a cylindrical microwave cavity, the resonant frequencies may not occur at equally spaced multiples of the fundamental frequency. They are then called overtones instead of harmonics. There may be several such series of resonant frequencies in a single resonator, corresponding to different modes of vibration.
Electromagnetic An electrical circuit composed of discrete components can act as a resonator when both an inductor and capacitor are included. Oscillations are limited by the inclusion of a resistor, which will be present, even if not specifically included, due to the resistance of the inductor windings. Such resonant circuits are also called RLC circuits after the circuit symbols for the components.
A distributed-parameter resonator has capacitance, inductance, and resistance that cannot be isolated into separate lumped capacitors, inductors, or resistors. An example of this, much used in filtering, is the helical resonator.
A single layer coil (or solenoid) that is used as a secondary or tertiary winding in a Tesla coil or magnifying transmitter is also a distributed resonator.
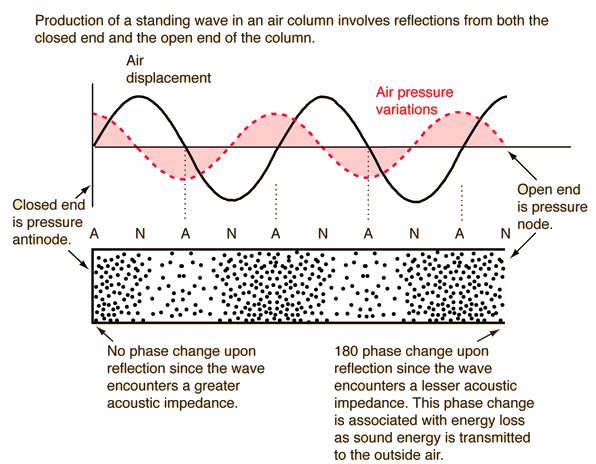
Cavity resonatorsA cavity resonator is a hollow conductor blocked at both ends and along which an electromagnetic wave can be supported. It can be viewed as a waveguide short-circuited at both ends (see Microwave cavity).
The cavity has interior surfaces which reflect a wave of a specific frequency. When a wave that is resonant with the cavity enters, it bounces back and forth within the cavity, with low loss (see standing wave). As more wave energy enters the cavity, it combines with and reinforces the standing wave, increasing its intensity.
Examples The cavity magnetron is a vacuum tube with a filament in the center of an evacuated, lobed, circular cavity resonator. A perpendicular magnetic field is imposed by a permanent magnet. The magnetic field causes the electrons, attracted to the (relatively) positive outer part of the chamber, to spiral outward in a circular path rather than moving directly to this anode. Spaced about the rim of the chamber are cylindrical cavities. The cavities are open along their length and so connect the common cavity space. As electrons sweep past these openings they induce a resonant high frequency radio field in the cavity, which in turn causes the electrons to bunch into groups. A portion of this field is extracted with a short antenna that is connected to a waveguide (a metal tube usually of rectangular cross section). The waveguide directs the extracted RF energy to the load, which may be a cooking chamber in a microwave oven or a high gain antenna in the case of radar.
,-14.06.2007.jpg/250px-Aust.-Synchrotron,-RF-Cavities-of-Linac-(Bunchers),-14.06.2007.jpg)
RF cavities in the linac of the Australian Synchrotron are used to accelerate and bunch beams of electrons; the linac is the tube passing through the middle of the cavity The klystron, tube waveguide, is a beam tube including at least two apertured cavity resonators. The beam of charged particles passes through the apertures of the resonators, often tunable wave reflection grids, in succession. A collector electrode is provided to intercept the beam after passing through the resonators. The first resonator causes bunching of the particles passing through it. The bunched particles travel in a field-free region where further bunching occurs, then the bunched particles enter the second resonator giving up their energy to excite it into oscillations. It is a particle accelerator that works in conjunction with a specifically tuned cavity by the configuration of the structures. On the beamline of an accelerator system, there are specific sections that are cavity resonators for RF.

An illustration of the electric and magnetic field of one of the possible modes in a cavity resonator
The reflex klystron is a klystron utilizing only a single apertured cavity resonator through which the beam of charged particles passes, first in one direction. A repeller electrode is provided to repel (or redirect) the beam after passage through the resonator back through the resonator in the other direction and in proper phase to reinforce the oscillations set up in the resonator.
In a laser, light is amplified in a cavity resonator which is usually composed of two or more mirrors. Thus an optical cavity, also known as a resonator, is a cavity with walls which reflect electromagnetic waves (light).
This will allow standing wave modes to exist with little loss outside the cavity.
Analogue television broadcasting MechanicalMechanical resonators are used in electronic circuits to generate signals of a precise frequency. These are called piezoelectric resonators, the most common of which is the quartz crystal. They are made of a thin plate of quartz with metal plates attached to each side, or in low frequency clock applications a tuning fork shape. The quartz material performs two functions. Its high dimensional stability and low temperature coefficient makes it a good resonator, keeping the resonant frequency constant. Second, the quartz's piezoelectric property converts the mechanical vibrations into an oscillating voltage, which is picked up by the plates on its surface, which are electrically attached to the circuit. These crystal oscillators are used in quartz clocks and watches, to create the clock signal that runs computers, and to stabilize the output signal from radio transmitters. Mechanical resonators can also be used to induce a standing wave in other medium. For example a multiple degree of freedom system can be created by imposing a base excitation on a cantilever beam. In this case the standing wave is imposed on the beam [1]. This type of system can be used as a sensor to track changes in frequency or phase of the resonance of the fiber. One application is as a measurement device for dimensional metrology[2].
AcousticThe most familiar examples of acoustic resonators are in musical instruments. Every musical instrument has resonators. Some generate the sound directly, such as the wooden bars in a xylophone, the head of a drum, the strings in stringed instruments, and the pipes in an organ. Some modify the sound by enhancing particular frequencies, such as the sound box of a guitar or violin. Organ pipes, the bodies of woodwinds, and the sound boxes of stringed instruments are examples of acoustic cavity resonators.
AutomobilesThe exhaust pipes in automobile exhaust systems are designed as acoustic resonators that work with the muffler to reduce noise, by making sound waves "cancel each other out"[1]. The "exhaust note" is an important feature for many vehicle owners, so both the original manufacturers and the after-market suppliers use the resonator to enhance the sound. In 'tuned exhaust' systems designed for performance the resonance of the exhaust pipes is also used to 'pull' the combustion products out of the combustion chamber quicker.
Percussion instrumentsIn many keyboard percussion instruments, below the centre of each note is a tube, which is an acoustic cavity resonator, referred to simply as the resonator. The length of the tube varies according to the pitch of the note, with higher notes having shorter resonators. The tube is open at the top end and closed at the bottom end, creating a column of air which resonates when the note is struck. This adds depth and volume to the note. In string instruments, the body of the instrument is a resonator.
The tremolo effect of a vibraphone is obtained by a mechanism which opens and shuts the resonators.
Stringed instrumentsString instruments such as the bluegrass banjo may also have resonators. Many five-string banjos have removable resonators, to allow the instrument to be used with resonator in bluegrass style, or without in folk music style. The term resonator, used by itself, may also refer to the resonator guitar.
The modern ten-string guitar, invented by Narciso Yepes, adds four string resonators to the traditional classical guitar. By tuning these resonators in a very specific way (C, Bb, Ab, Gb) and making use of their strongest partials (corresponding to the octaves and fifths of the strings' fundamental tones), the bass strings of the guitar now resonate equally with any of the 12 tones of the chromatic octave. The Guitar Resonator is a device for driving guitar string harmonics by an electromagnetic field. This resonance effect is caused by a feedback loop and is applied to drive the fundamental tones, octaves, 5th, 3th to an infinite sustain.
Romero Mora Loren A. C.I:18762881
Tomado de:
http://en.wikipedia.org/wiki/ResonatorCRF













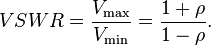

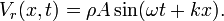
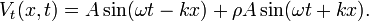











 , the length of a round trip is
, the length of a round trip is  . In order to cause resonance, the phase of a sinusoidal wave after a round trip has to be equal to the initial phase, so the waves will reinforce. So the condition for resonance in a resonator is that the round trip distance,
. In order to cause resonance, the phase of a sinusoidal wave after a round trip has to be equal to the initial phase, so the waves will reinforce. So the condition for resonance in a resonator is that the round trip distance,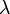 of the wave:
of the wave:

,-14.06.2007.jpg/250px-Aust.-Synchrotron,-RF-Cavities-of-Linac-(Bunchers),-14.06.2007.jpg)








